Hide Table of Contents

Rayleigh Damping

The  n x n symmetric damping matrix [C] is formulated as a linear combination of the mass [M] and stiffness [K] matrices:

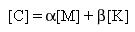(Equation 1)

The type of damping described by (Equation 1) is known as Rayleigh or proportional damping.

This form of [C] is orthogonal with respect to the system eigenvectors.

By applying the modal coordinate transformation, the modal damping matrix [c] becomes diagonal:

(Equation 2)

You can define Rayleigh damping for linear and nonlinear dynamic studies.

Relation of Rayleigh Coefficients and Modal Damping Ratio

The modal damping matrix [c] is given by:

(Equation 3)

The coefficient of viscous damping ci for the ith mode is calculated by:

(Equation 4),

and the viscous damping ratio zi is expressed as

(Equation 5)

If the damping ratios for the ith and jth modes are z i and z j, then the Rayleigh coefficients a and b are calculated from the solution of the two algebraic equations:

(Equation 6)

If both modes have the same damping ratio ( z i = z j = z) , then the values of a and b are given by:

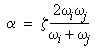(Equation 7)   (Equation 8)

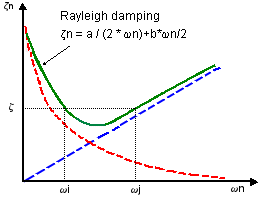
The viscous damping ratio z for any other mode varies with frequency as shown in the figure:

Related topics

Global Damping



Provide feedback on this topic

SOLIDWORKS welcomes your feedback concerning the presentation, accuracy, and thoroughness of the documentation. Use the form below to send your comments and suggestions about this topic directly to our documentation team. The documentation team cannot answer technical support questions. Click here for information about technical support.

* Required

 
*Email:  
Subject:   Feedback on Help Topics
Page:   Rayleigh Damping
*Comment:  
*   I acknowledge I have read and I hereby accept the privacy policy under which my Personal Data will be used by Dassault Systèmes

Print Topic

Select the scope of content to print:
x

We have detected you are using a browser version older than Internet Explorer 7. For optimized display, we suggest upgrading your browser to Internet Explorer 7 or newer.

 Never show this message again
x

Web Help Content Version: SOLIDWORKS 2012 SP05

To disable Web help from within SOLIDWORKS and use local help instead, click Help > Use SOLIDWORKS Web Help.

To report problems encountered with the Web help interface and search, contact your local support representative. To provide feedback on individual help topics, use the “Feedback on this topic” link on the individual topic page.

0.20.20