Description
An 80" long beam has a square cross-section of 2" X 2". A block of 8" X 2" x 6" is attached to the beam as shown below. Determine the natural frequency of the system for simply supported and fixed end restraints. In separate studies, the block is modeled as a remote mass and as a solid.
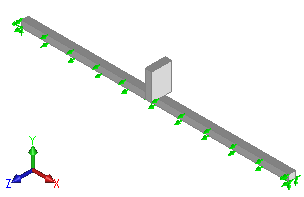
File Name
Browse to
drive letter:\Users\Public\Public
Documents\SOLIDWORKS\SOLIDWORKS
version\samples\Simulation
Examples\Verification\Frequency_16.SLDASM and open the
file.
Material Properties
Modulus of elasticity = 10,007,604 psi, Poisson's ratio = 0.33, Density = 0.0975437 lb/in3.
Results
Four studies are created:
- Study Fixed-Fixed models the block as a remote mass
- Study Pinned-Pinned models the block as a remote mass
- Study Solid-Fixed-Fixed models the block as solid
- Study Solid-Pinned-Pinned models the block as solid
The fundamental frequency for a slender beam with concentrated center mass is:
f1 = (4/π)*(3 E I /L3 (M+0.37 Mb) )1/2 (fixed-fixed end restraints)
f1 = (2/π)*(3 E I /L3 (M+0.49 Mb) )1/2 (pinned-pinned end restraints).
where:
- E: Modulus of elasticity
- I: Area moment of inertia of beam (neutral axis)
- M: Mass
- Mb: Mass of beam
- L: Span of beam
| |
Fundamental Frequency, Hz
(Fixed-Fixed) |
Fundamental Frequency, Hz
(Pinned-Pinned) |
| Theory
|
48.37 |
22.27 |
| SOLIDWORKS Simulation (block as solid) |
48.80 |
22.58 |
| SOLIDWORKS Simulation (block as remote mass)
|
49.13 |
22.75 |
Reference
Robert D. Blevins, "Formulas for Natural Frequency and Mode Shape", Krieger Publishing Company, Florida, Reprint Edition 2001, pp. 158-159.