Description
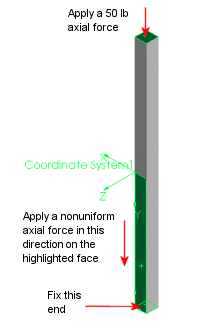
A 20"-uniform bar of 1"X1" square cross section, made of Alloy Steel, is fixed at one end and subjected to an axial load of magnitude P=50 lbs at the other free end. Another nonuniform axial load that varies linearly from a value of p=10 lbs at the fixed end to a zero value at a distance a=10" from the fixed end is also applied. Assuming that P does not cause buckling on its own, calculate the value of the product (p*a)' that will cause buckling.
File Name
Browse to drive
letter:\Users\Public\Public Documents\SOLIDWORKS\SOLIDWORKS
version\samples\Simulation
Examples\Verification\Buckling_4.SLDPRT and open the
file.
Modeling Hints
A coordinate system (Coordinate System1) was created at the middle point of the left edge of the bar to define the nonuniform axial force. Both axial loads are acting along plane Dir 2.
Results
The following equation calculates the product (p*a) that will cause buckling: (p*a)' = Kπ2 E I/L, where (p*a)' is the theoretical critical value of the product (p*a), K is an empirical factor that depends on the ratio a/L and P/(p*a). The value of K is looked up from table 34 of the reference cited below as 0.49. E, I, and L are the Elasticity modulus of Alloy Steel, the moment of inertia of the cross section of the bar, and the length of the bar respectively.
| |
Analytical Solution |
SOLIDWORKS Simulation |
| Buckling value (p*a), lbs |
30680 |
31200 |
Reference
Roark and Young, "Formulas for Stress and Strains," Fifth Edition, McGraw-Hill Book Company, Chapter 14, pp 540.