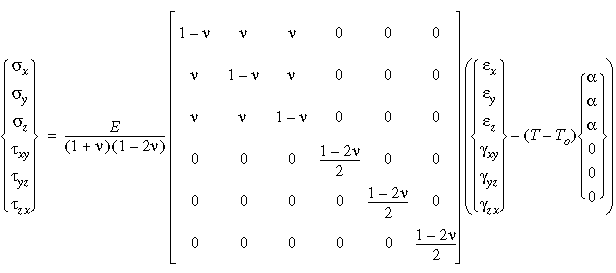A material is called isotropic if its properties do not vary with
direction.
Isotropic materials have identical elastic modulus, Poisson's ratio, coefficient of
thermal expansion, thermal conductivity, etc. in all directions. The term isothermal is
sometimes used to denote materials with no preferred directions for coefficients of
thermal expansion.
In order to define the isotropic elastic properties, you must define the elastic
modulus E. The program assumes a value of 0.0 for Poisson's ratio ν, if no specific
value is specified. A common value for the Poisson's ratio is 0.3.
The shear modulus G is calculated internally by the program from the
formula:
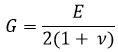
You do not have to enter a value for a shear modulus for a linear elastic isotropic
material. If you enter a value for the shear modulus, the program ignores it and
calculates the shear modulus from the above formula.
The stiffness matrix for an isotropic material contains only two independent coefficients. The following sections describe the isotropic stress-strain relations in two- and three-dimensions including the effect of thermal strains.
The most general form of the isotropic stress-strain relations including thermal effects is shown below: