Hyperelastic Mooney - Rivlin Model

The Mooney-Rivlin strain energy density function is expressed as:

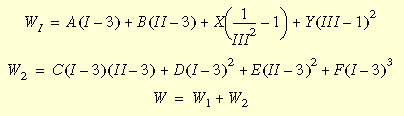
where I, II, and III are invariants of the right Cauchy-Green deformation tensor and can be expressed in terms of principal stretch ratios; A, B, C, D, E, and F are Mooney material constants, and

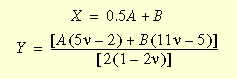
It has to be noted that as the material approach incompressibility, the third invariant, III, approaches unity, while Y approaches infinity. Thus, for values of Poisson's ratio close to 0.5, the last term in w1 remains bounded, and a solution can be obtained.

The Mooney-Rivlin material model can be used with solid elements and thick shells. The material properties for Mooney-Rivlin model are input in the Material dialog box.  Up to six Mooney-Rivlin constants can be input: Mooney_A, Mooney_B, Mooney_C, Mooney_D, Mooney_E, and Mooney_F.

The Mooney-Rivin constants are automatically computed when the Use curve data to compute material constants option in the Material dialog box is checked. The constants are saved in a text file with the extension .log in the active result's folder for the study.

NOTES

• Use the NR (Newton-Raphson) iterative method.

• Values of Poisson's ratio greater than or equal to 0.48 but less than 0.5 are acceptable. When the displacement-pressure formulation is used, Poisson's ratio is recommended in the range from 0.499 to 0.4999.

• Rubber-like materials usually deform rapidly at low magnitudes of loads thus requiring a slow initial loading.

• When dealing with rubber-like materials, due to the highly nonlinear behavior of the problem, rapid increase in loading will often result in either numerical instability (negative diagonal terms in the stiffness) or divergence during equilibrium iterations. The automatic-adaptive stepping algorithm can help in such cases.

• The displacement or the arc-length control may prove to be more effective than force control when negative diagonal terms repeatedly occur under various loading rates.

• For shell elements with thick formulation, the analysis is simplified since incompressibility does not result in unbounded terms. The formulation is derived assuming perfect incompressibility (Poisson's ratio of 0.5), and therefore NUXY is neglected.

• Constants A and B must be defined such that (A+B) > 0. For more information about how to determine the values of the A and B constants, refer to the work by Kao and Razgunas.

To define hyper elastic models...