Plasticity Tresca Model
This criterion is based on the assumption that in the state of yielding, the maximum shearing stress at all points of a medium is the same, and is equal to half of the yield stress that is obtained from a uniaxial tension test for the given material.
In the three-dimensional case, yield occurs if at least one of the inequalities is satisfied:

In other words, yielding is based on the maximum shearing stress which is equal to half the difference between the maximum and minimum principal stresses. Based on this criterion, the intermediate principal stress does not influence the state of yielding.
Note: In some design codes and references, the Tresca equivalent stress is defined as twice the maximum shear stress which is equal to (σ1 –σ3), or else the stress intensity.
Shearing Stress Intensity
The shearing stress intensity is defined by the square root of the second invariant of the stress deviator and can be expressed as:
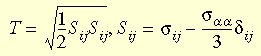
State of Pure Shear
The state of pure shear is defined as:

For this state, the shearing stress intensity and the maximum shearing stress are equivalent:
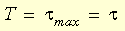
Using the Tresca conditions the shearing stress at the yield point is obtained to be half of the tensile yield stress:

Based on the von Mises yield criterion the shearing yield stress is equivalent to:
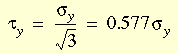
Notes
-
Both isotropic and kinematic hardening rules are available for the Tresca model. A linear combination of isotropic and kinematic hardening is implemented when both the radius and the center of yield surface in deviatoric space can vary with respect to the loading history.
-
The parameter RK defines the proportion of kinematic and isotropic hardening.
-
For pure isotropic hardening, the parameter RK has the value 0. The radius of the yield surface expands but its center remains fixed in deviatoric space.
-
For pure kinematic hardening, the parameter RK has the value 1. The radius of the yield surface remains constant while its center can move in deviatoric space.'
Comparison of Tresca and von Mises Criteria for Plasticity